ESTIMATING

 ESTIMATING 

What is an Estimate? 

    Before starting any work for it’s execution the owner or client or builder or contractor should have a thorough knowledge about the cost of the work that can be completed within the limits of his found or probable cost that may be required to complete the work. It becomes therefore necessary to prepare the probable cost or estimate for the intended work from its plan and specification. Otherwise, it may so happen that the work has to be stopped before its completion due to shortage of funds or materials. In this reasons the above an estimate for any public construction work is asked to be prepared and submitted previously so that sanction of necessary funds may be obtained from the authority concerned.

    Thus an estimate for any construction work may be defined as the process of calculating the quantities and cost of the various items required in the work. To prepare an estimate, drawings consisting the plan, the elevation and the sections through important points, along with a detailed specification giving specific description of all workmanship, properties and proportion of materials, are required.         

Different Types of Estimates.

    An estimate prepared from the plans and specifications and consulting the current market prices of materials is never the actual cost of work. Because the cost of materials and labour may vary during the period of its actual execution or due to variations and modifications of actual dimensions shown in the drawing or due to some unforeseen contingencies. The difference between the estimated and actual cost will depend upon the skill and accuracy of estimator. There are different types of estimates and they are

1.     1. A Detailed Estimate.

2.      2.  A Preliminary or approximate or rough estimate.

3.      3. A Quantity estimate or quantity Survey.

4.    4. Revised estimate.

5.    5. A Supplementary Estimate.

6.    6. A Complete Estimate.

7.    7. Annual Maintenance Estimate.

A Detailed Estimate: This includes the quantities and cost of everything required for satisfactory completion of work and this is the best of most reliable and mostly used that can be made. A detailed estimate is accompanied with (a) Report (b) Specifications. (c) Detailed drawing showing plans different sections, layout plan. Key or Index plan etc.. (d) Design data and calculations. (e) Basis of rate adopted in the estimate. Such a detailed estimate is prepared for technical sanction, administrative approval and also to execute a contract with the contractor. The method of preparation a detailed estimate has been in another article.

A Preliminary or approximate or rough estimate: This is an approximate estimate made to findout an approximate cost in a short time and  thus enable the responsible authority concern to consider the financial aspect of the scheme for according sanction to the same. Such an estimate is framed after knowing the rate of similar works and by the use of any one of the following methods of estimate:-

a.  (a)  Unit rate estimate.

b.  (b) Plinth area estimate.

c.   (c) Cube rate estimate.

 

a.   Unit rate estimate: In this method all costs of a unit quantity such as per k.m. for a highway per metre, of span for a bridge, per classroom for school building, per bed for hospital, per litre for water tank etc. are considered first and the estimate is prepared by multiplying the cost per corresponding unit by the number of units in the structure.

 

b.   Plinth area estimate: In this method plinth area should be calculated by taking the external dimensions of the building at the plinth. Court area and other open areas should not be included in the plinth area. At the beginning, when plan of a building has not yet been prepared or available determine the total floor area of all the rooms corridor, verandh, kitchen, W.C. and bath according to the requirement of the client, and of the total area thus found, may be added for walls and waste to get the approximate total plinth area. The plinth area thus found shall be multiplied by the plinth area rate for similar type design and specification of building at the locality.

 

c.   Cube rate estimate: The method of estimating building cost by the cubic metre (or cubic foot) of volume is more accurate in general, than the method of plinth area method. Because cost of building depends not only on plinth area but also on their respective height. The best of estimating cost by the cubic rate is to find the volume of the building (length x breadth x height) and then multiply the volume by the local cubic rate for similar type of building. Length and breadth should be measured external to external excluding plinth offset, corbelling, string course etc. The height should be measured from the top of the flat roof (or half way of the sloped roof) to half the depth of the foundation below the plinth. Parapet is not to be included.

A Quantity estimate or quantity Survey: This is the complete estimate of the quantities of materials that may be required to complete the work concerned.

 Revised estimate: When a sanctioned estimate is likely to be exceeded by more than 5 percent either from the rates being found insufficient due to change to price level or from any cause whatever, except important structural alterations an estimate is prepared which is called a revised estimate. In case where important structural alterations are contemplated through not necessarily involving an increased outlay revised estimate should also be submitted for technical sanction. The method of preparation a revised estimate is same as the detailed estimate. A cooperative statement showing in an abstract from the probable variation or deviation of each item of work, its quantity, rate as compared with the original estimate stating the reasons of variations or deviation should be attached with it.    

A Supplementary Estimate: While a work is in progress some additional works may be thought necessary for development of a project which was not foreseen when the original estimate was prepared and the expenditure for such supplementary work cannot be meet up from savings elsewhere within the Grant, an estimate is then prepared to cover up all such works which is known as supplementary estimate. The method of preparation of a supplementary estimate is same as that a detailed estimate and it should be accompanied by a full report of the circumstances which render it necessary. The abstract must the supplementary amount.

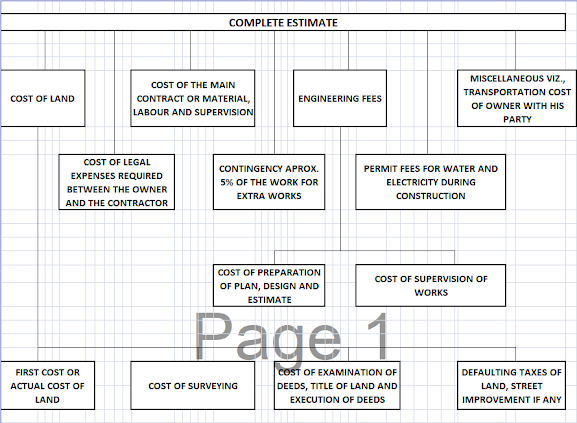
 A Complete Estimate: This is an estimated cost of all items which are related to the work in addition to the main contract or to the detailed estimate.

   One may think that an estimate of a structure includes only the cost of land and the cost of the main contracts or labour, materials and supervision. But there are many other cost items to be included. A picture of a complete estimate is diagrammatically shown as below.  

Annual Maintenance Estimate: After completion of a work it becomes necessary to maintain the same for its proper function and for the same estimate is prepared for the items which require renewal, replacement, repairs etc. in the form of a detailed estimate.

 

 

NEXT POST ON “HOW TO PREPARE DETAILED ESTIMATE”  

FORMULA FOR CLCULATION OF VOLUME

 

FORMULA FOR CLCULATION OF VOLUME

 

D = Common distance between sections.

 Trapezoidal rule or average end area rule.

 

Volume (Cutting or Filling),

 

                           V = D/2[A₁ + An + 2(A₂ + A₃ + A₄ + … +An-₁)]

 

i.e.   Volume = Common distance {Area of 1^st section + Area of last section

                                  2              

                                                + 2(Sum of area of other sections)}

 

Prismoidal rule.

 

Volume (Cutting or Filling),

 

          V = D/3[(A₁+An) + 4(A₂+A₄+...+ An-₂) + 2(A₃+A₅+... + An-₁)]

 i.e.   Volume = Common distance {Area of 1^st section + Area of last section

                                  3              

                                                 + 4(Sum of area of even sections)

                                                 + 2(Sum of area of odd sections)}

 

This formula is applicable when there are an odd number of sections.

 

PRISMOIDAL CORRECTION FOR TRAPEZOIDAL OR AVERAGE END AREA RULE

 

i.             Prismoidal correction for level section:

 

            Cp = D x s (h – h)²    (Considering, transverse slope= 1 in n side slope= S : 1)

                        6       

 

 

 

 

ii.            Prismoidal correction for two level section:

 

            Cp = D x s    n²      x (h₁ – h₂)²

                        6    n² - s     

 

iii.          Prismoidal correction for three level section:

 

            Cp = D  (h₁ – h₂)    (Whole width of 1^st section – Whole width of 2^nd section)

                           12

 

iv.          Prismoidal correction for Side-hill two level or Part cut-part fill:

 

(a)        Cp (for cutting) =      D       x n²(h₁ – h₂)²    (side slope= s₁ : 1)

                                              12(n – s₁)

 

(b)        Cp (for filling) =      D       x n²(h₁ – h₂)²    (side slope= s₂ : 1)

                                           12(n – s₂)

NEXT POST ON ESTIMATING

COMPUTATION OF VOLUME

 

VOLUME

Earthwork operations involve the determination of volumes of material that is to be excavated or embanked in engineering project to bring the ground surface to a predetermined grade. Volumes can be determined via cross-sections, spot levels or contours. For computation of the volume of earth work, the sectional areas of the cross-section which are taken transverse to the longitudinal section during profile leveling ate first calculated. Again, the cross-sections may be different types, namely: (a) Level (b) Two-level, (c) Three-level, (d) Side-hill two level or Part cut-part fill and (e) Multi-level.

After calculation of cross-sectional areas, the volume of earth work is calculated by:

i.             The trapezoidal rule or average end area rule.

ii.            The prismoidal rule.

 

1)   The prismoidal rule gives the correct volume directly.

2)   The trapezoidal rule does not give the correct volume. Prismoidal correction should be applied for this purpose. This correction is always subtractive.

3)   Cutting is denoted by a positive sign and filling by a negative sign.

A. Level Section

 

When the ground is level along the transverse direction

Cross-sectional area = (b + b + 2sh) x h

                                         2

                              = (b + sh)h

B. Two-Level Section

 

When the ground surface has a transverse slope:

 

PB = b/2

Bx = sh₁

b₁ = b/2 + sh₁                   …………………………………………. (a)

Ee = (h₁ – h)

b₁ = n x Ee = n(h₁ - h)       …………………………………….…….(b)

 

From (a) and (b),       b/2 + sh₁ = n(h₁ - h)

 

                     Or        h₁(n – s) = n(h + b/2n)

  

                     Or        h₁ =     n      x (h + b/2n)       ….…(1)

                                        (n – s)

 

From (2) and (a),       b₁ = b +    ns      x (h + b/2n)  ……(2)

                                        2    (n – s)

 

                                h₂ =     n      x (h - b/2n)        . ……(3)

                                        (n + s)

 

                                b₂ = b +    ns      x (h - b/2n)   ……(4)

                                        2    (n + s)

Area ABCDE = ∆DOE + ∆COE - ∆AOB

                   = 1 OE X Dd + 1 OE x Ce - 1 AB x OP

                       2                 2                2

 

Here,      OE = OP + PE =  b + h

                                      2s

Dd = b₂        Ce = b₁

 

AB = b          OP =  b

                             2s

 Area = ½{( b + h)b₂ + ½( b + h)b₁ - 1b x b}

                     2s                      2s              2     2s

 

        = ½{( b + h)(b₁ + b₂) – b²}       ……(5)

                     2s                           2s            

 

 

 C. Three-Level Section

 

When the transverse slope is not uniform:

 

  Area ABCOD = ∆DOP + ∆COP + ∆DAP + ∆BCP

 

                     = 1 x h x b₂ + 1 x h x b₁ + 1 x b x h₂ + 1 x b x h₁

                         2                 2                2    2           2    2

 

                Area = {1/2(b₁ + b₂) + b/4(h₁ + h₂)}

 

Here               h₁ = OP + Oe = h + b₁/n₁

 

                      h₂ = OP – ef = h – b₂/n₂         

Deduction of formula for b₂ and b₁

                       b₂ = AP + AK = b/2  + sh₂

 or,                   h₂ = {b – (b/2)}                        … (1)

                                     s

Also

                       b₂ = ef x n₂ = (h – h₂)n₂

or,                   h₂ = hn₂ – b₂                              … (2)

                                  n₂

From (1) and (2)

 

                      {b – (b/2)} = hn₂ – b₂                     

                              s₂                n₂

 

                     b₂n₂ – bn₂/2 = hn₂s - b₂s

  

                    b₂(n₂ + s) = n₂(sh + b/2) = n₂s(h + b/2s)

 

                    b₂ = n₂s   x (h + b/2s)

                           n₂ + s

Similarly,

 

                    b₁ = n₁s   x (h + b/2s)

                           n₁ - s

 

 

D. Side-hill two level or Part cut-part fill

 

When the ground surface has a transverse slope, but the slope of the ground cuts the formation level partly in cutting and partly in filling, the following method is adopted:

 

Here,          h =  n   x (b/2n + h)

                      n – s

                  b₁ = b +    ns      x (h + b/2n)

                         2    (n – s) 

Then h₂ and b₂ are deduced as follows:

                            b₂ = b + AA' = b + sh₂                     … (i)

                                    2             2

Again                     b = EE' = O'E' x n = (h + h₂)n           … (ii)

 

From (i) and (ii),     b + sh₂ = (h + h₂)n 

                             2

 

Or                          h₂(n – s) = b – hn = n  b – h

                                               2               2n

 

                              h₂ =  n   x (b/2n - h)

                                    n – s

 

 From (i)                               b₂ = b +    ns      x (b/2n - h)

                                                    2    (n – s)

Area in Cutting:

     Area of ∆PBC,

 

                         A₁ = 1 x PB x h₁

                                 2

Here,                 PB = OB + OP = b + nh

                                                  2

 

                       A₁ = 1  x   b + nh     n     x  b + h  

                                2      2            n – s    2n

 

                          

                             = 1   b + nh     1    x b + nh

                                 2   2           n – s   2

 

                             = 1   {(b/2) + nh}²

                                 2        n – s

 

Area in Filling:

     Area of ∆APE,

 

                         A₂ = 1 x PA x h₂

                                 2

 

Here,                 PA =  b - nh

                                  2

 

                        A₂ = 1  x   b - nh      n     x  b - h  

                                2      2            n – s    2n

 

                          

                             = 1   b - nh      1    x  b - nh

                                 2   2           n – s   2

 

                             = 1   {(b/2) - nh}²

                                 2        n – s

In the above case, the side slopes for cutting and filling are assumed to be equal. But in actual practice, the side slope of cutting is different from that of filling. Let the side slop of cutting be s₁ : 1.

   Then,

 

                                 b₁ = b +    ns₁      x (h + b/2n)

                                         2    (n – s₁)

Area in cutting,

 

                                 A₁ = 1   {(b/2) + nh}²

                                         2        n – s

E. Multi-level Section

 The cross-sectional data pertaining to an irregular section are noted in the following form:

 

Left	Centre	Right
±h₂/2            ±h₁/2	±h/0	±h₃/b₃            ±h₄/b₄

 

A positive sign in the numerator denotes a cut, and a negative sign indicates a fill.

 

The denominator denotes corresponding horizontal distance from the centre. Starting from the centre (E) and running outwards to the right and left, the coordinates of the vertices are arranged, irrespective of algebraic sign, in determinant form:

 

 

A        G        F        E        D       C         B             

 

0₀      h₁       h₂       h₃       h₄      h₅       0              

b/2     b₁       b₂       0        b₄      b₅      b/2

 

The sum of products of the coordinates joined by the solid line is

 

       ∑ P = h₃ x 0 + h₄ x b₃ + 0 x b₄ + h₁ x 0 + h₂ x b₁ + 0 x b₂          

 

The sum of products of the coordinates joined by the dotted line is

 

       ∑ Q = h x b₃ + h₃ x b₄ + h₄ x (b/2) + h x b₁ + h₁ x b₁ + h₂ x (b/2)

 

Area = ½ (∑ P - ∑ Q)         

 

(Next post on “FORMULA FOR CLCULATION OF VOLUME”)